Magnetic Excitations & Geometric Confinement:
Theory and Simulations
Gary Matthew Wysin - Kansas State University, U.S.A.
Published December, 2015, as an
IOP Expanding Physics ebook
This book gives a description of some types of underlying order and dynamics in magnetic materials under various geometrical constraints, such as the shape of the system, geometrically structured microscopic interactions, due to different lattice structures, and also fundamentally anisotropic magnetic forces. It starts from a review of fundamental principles of magnetostatics and describes the use of classical spin mechanics and micromagnetics for finding the dynamical magnetic excitations in various model magnetic systems. It is intended for physics, chemistry and other science students from the upper undergraduate to the graduate level, in physics, engineering and related fields.
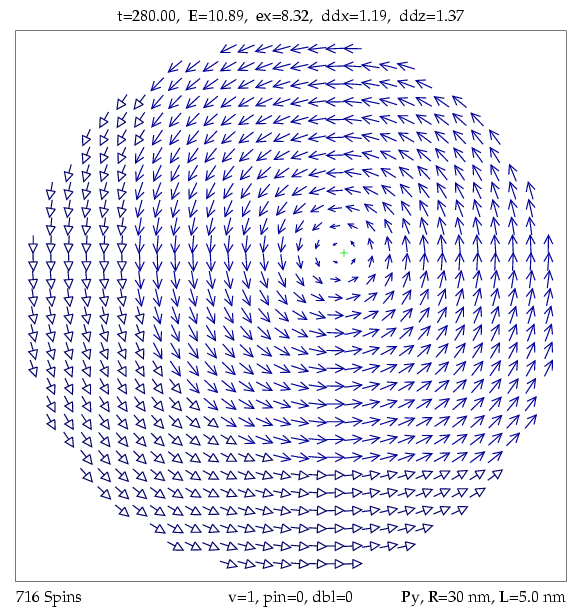
Typical magnets of confined geometry support not only linear spin wave excitations but also nonlinear excitations such as solitons and vortices, that can behave in certain aspects like particles. The particle-like properties are analyzed, as well as the dynamics of these objects under applied fields. For vortices in nano-sized thin disks, the effect of temperature can be to drive their natural dynamics, which offers an interesting system for study. Models of artificial spin ice also provide a challenge for describing effects of frustration on their dynamics.